# 54 | M^ZERO, Singleness of Money, and Proofs By Contradiction
Centonarius (I): Thoughts Worth More Than the Space They Take

MˆZERO LABS_
On the 5th of April a project named M^ZERO announced the completion of a $22.5m funding round, participated by some of the most relevant investors in blockchain technology.
The funds would allow the team to build a decentralised value transmission mechanism that could connect institutional-like agents to exchange and compose value on-chain. Sitting on top of the Ethereum distributed computing layer, the M^ZERO financial middleware would provide parties with the ability to develop an infinite number of financial use-cases, from the most simple (new and better forms of money) to the most sophisticated—new and better forms of financial aggregates and derivatives.
As co-founder and CEO of M^ZERO Labs, the team researching the M^ZERO open-source protocol, I am aware that many design and business decisions have been informed by my own research, to which Dirt Roads has been a window for almost two years. It goes without saying, however, that what I intend to debate here on DR has represented (and will represent) solely my independent judgement on topics related to value transmission, and does not in any way relate to the company’s or any investor’s views.
The above is a necessary caveat to preserve the intellectual independence and honesty of a researcher, alongside the managerial duties of a CEO. Without such independence and honesty, a project like Dirt Roads would better cease to exist. As I have done in the past, I intend to make my opinions, as well as any potential conflict of interest, as transparent as possible—I trust the reader will help me keep this promise with respect and with a presumption of good faith.
On the Singleness of Money
On the 11th of April, the Bank for International Settlements (BIS) issued a bulletin on the evolution of different classes of tokens linked to fiat currencies, focusing on the debate between stablecoins and tokenised deposits. While the swiftness of the conclusions suggest, to me at least, a narrow (and many might say biased) scope in analysing the evolution of money in its core characteristics, the document focuses the attention on core capabilities of money that any researcher of monetary design should take into consideration.
Singleness of money → Cornerstone of modern money functionality is the certainty that any type of money, being privately (i.e. bank deposits) or publicly (i.e. cash) issued, benefits from an exact and constant exchange rate within a sovereign monetary system. We have discussed this concept at length here on DR, reminding the reader of the several benefits that a smooth monetary system brings—especially for the guarantor. The paper uses this principle, i.e. the ability to structurally provide absolutely stable and predictable exchangeability within a currency system, as the guiding light to assess alternative approaches to the creation of tokenised money instruments.
(Asset-backed) stablecoins → Currently dominating stablecoin models (read $USDC/ Tether) represent financially a transferrable claim on the issuer—Circle in the case of $USDC. Independently from the degree of creditworthiness of the issuer, this model brings uncertainty into the system in the form of mark-to-market volatility. This type of volatility can compound within a currency system creating frictions that, even if minimal, can jeopardise the functionality of money as a whole. Imagine, for example, if our lives would be exposed to the continuous mark-to-market of our bank deposits.
We have discussed at length here on DR Circle’s risk profile, just weeks before SVB’s unfolding (i.e. when Twitter influencers didn’t care) and had highlighted the unsatisfactory risk/ reward proposition of the instrument. Below an extract from issue #52—emphasis is mine.
Interestingly enough, Circle segregated deposits are not immune to all types of risk. Yes, we can assume that credit risk is negligible here from a dollar perspective—with pretty much all segregated deposits invested in US government securities, but the same cannot be said for counterparty risk. Due to the massive volumes intermediated by Circle and the limit in scope of deposit insurance, out of the c. $10b that Circle was holding in cash in regulated financial institutions in 2021—24% of the c. $42b total segregated value as per their reports, only a mere $1.75m (millions—0.02%) in aggregate was covered by FDIC deposit insurance. This means that if a bank goes under, $USDC holders go in line with other creditors for the cash portion of the stablecoin backing, which is indeed 24%. It is quite a lot of counterparty risk for receiving zero (0.00%, 0 bps) income as a $USDC holder. Nobody cared when the risk-free proxy was also at zero, but that reality is far away in the past.
Ironically, we had to wait only a few days for this scenario to materialise. In the middle of the SVB’s debacle Circle confirmed to the world their $3.3b exposure (out of c. $40b $USDC issued) to the bank and, as a consequence, the marking-to-market of $USDC vs. the dollar started fluctuating. Market dynamics exacerbated the phenomenon, bringing $USDC’s valuation down to 90c, probably overestimating the real counterparty risk by a factor of 10x—but that’s what markets do. At the same time, $USDT started trading at a premium over the dollar, suggesting a rotation from known unknowns into unknown unknowns—ironic but that’s what markets do too.
Tokenised deposits → The alternative to asset-backed stables proposed by the BIS (and by other financial institutions) are authorised non-bearer instruments, or in other words some sort of tokenised bank deposit. Differently from what happens for asset-backed stables, the exchange of a non-bearer instrument constitutes merely a change on the ledger of the (regulated) issuer, and eventually of those issuers with the centralised entity that connects them. In other words, a digitised deposit fully piggybacks on the current banking system. How does this work in a nutshell?
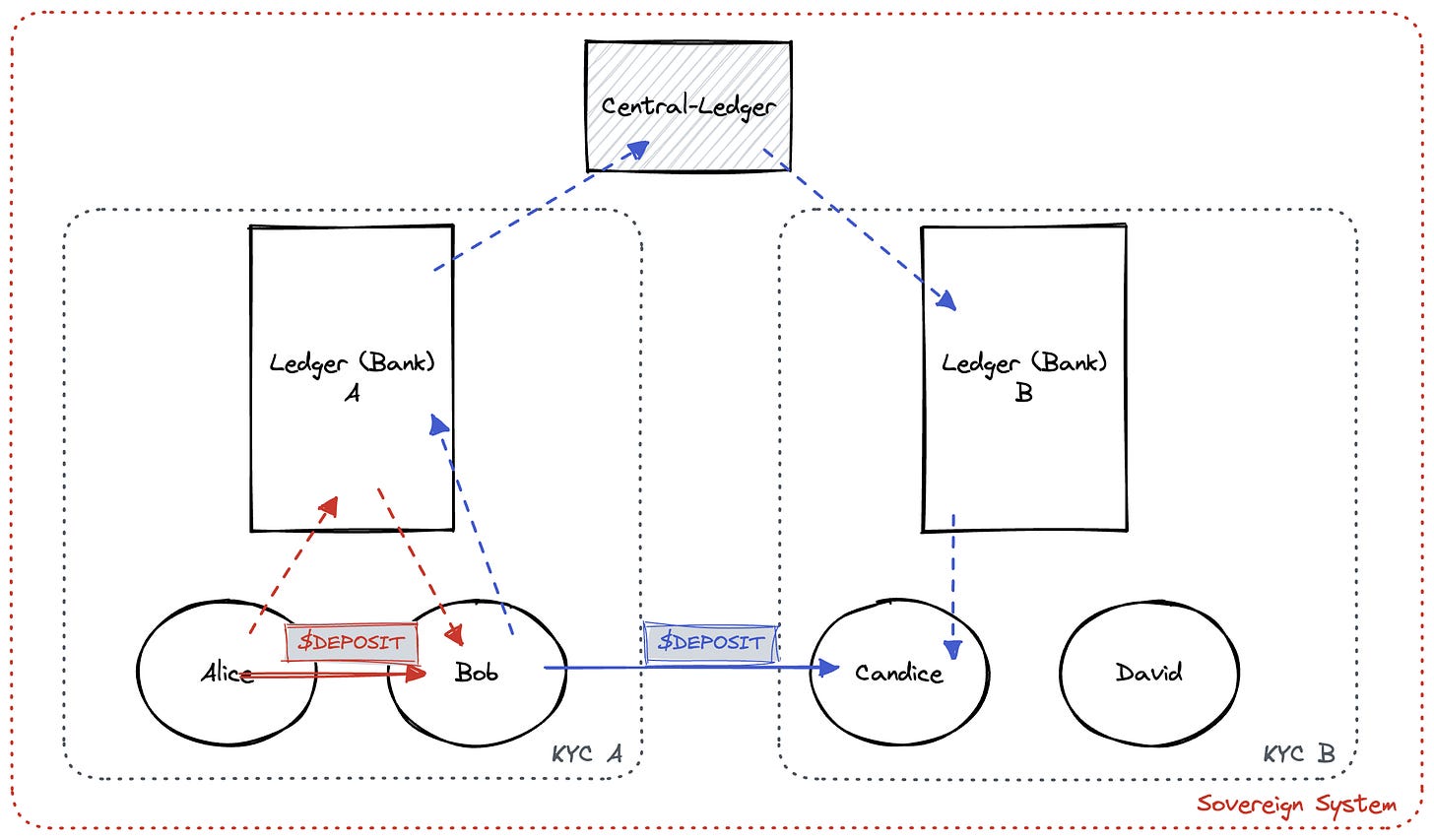
Alice sends money to Bob → Alice and Bob, who in the diagram below belong to the same KYC garden, want to exchange value through $DEPOSIT. In doing so they simply instruct Ledger A to update its own ledger for the transfer of value between them. There is no change in counterparty
Bob sends money to Candice → Bob and Candice, who do not belong to the same KYC garden, want to exchange value through $DEPOSITS. In doing so they are:
Instructing Ledger A to debit Bob and Ledger B to credit Candice
Instructing Ledger A and Ledger B to agree with Central-Ledger and update its own meta-ledger for the two institutions
Under the (strong) assumption that the meta-counterparty (Central-Ledger) provides total coverage of the underlying liabilities of its children, this approach should ensure singleness of money within the Sovereign System—meaning that Ledger A’s money is as good as B’s. Being singleness of money the key deciding factor in the BIS analysis, the paper concludes about the superiority of tokenised deposits vs. asset-backed instruments.
Here you go. It was so simple. I have many remarks over the conclusion of the BIS, but let me start with the ones below, in no particular order:
It is at least ironic that the source of most, if not all, the volatility disliked by the BIS and experienced by regulatory-friendly asset-backed stablecoins like $USDC hasn’t come from assets but rather from banks—i.e. from its exposure to the centrally guaranteed regulated banking system that the BIS treats as structurally superior from a counterparty risk perspective
In their current set up central banks/ regulators are far from guaranteeing the entirety of banks’ customers liabilities, with deposit insurance schemes being able to protect only retail savers—FDIC insurance is sybil resistant. Although limits exist to avoid full-blown moral hazard, they also have the vicious effect of protecting insiders who can get out of certain issuers before those issuers shut the doors to the rest, leaving most clients with nothing but praying for a bailout
While the volatility induced by mark-to-market of asset-backed stablecoins is not desired and can jeopardise the functionality of money, the uniqueness of money solved for by tokenised deposit instruments has many "subject to’s”: singleness is subject to each individual complying to institution-specific changing requirements, it is subject to each institution complying to central requirements, it is subject to each central authority complying to their own ever-changing mutual requirements. Tokenised deposits’ singleness is grandfathered singleness—or in other words nothing more than a functionality trick
It is also ironic that we need to read the whole BIS report (something that journalists seldom do) to get to the caveat provided by the BIS, i.e. that the singleness of money is a crucial characteristic for payment instruments, while it might not be the dominant characteristic for storage of value instruments—most of us use money to store value too
Personally, I am joining the BIS in affirming the inferiority of the current asset-backed design. I’d suggest the BIS, however, to expand their research scope of monetary design before claiming that, almost by an incredible coincidence, the current shape of the banking system is exactly the best possible set-up that money can ever achieve. But that’s for another day.
Proofs by Contradiction
Simple ideas are extremely powerful. Yet, they don’t sell books. Rather than just work within an existing system, simple ideas are bare, outrageous, expressions of an absolute truth we had for some reasons ignored but that now, after having been revealed to us, we cannot do anything to suffocate. Simple ideas often represent paradigmatic leaps, and in doing so they are resisted, intensely, by everyone, including their discoverers, who try to choke and hide them incapable of accepting what those ideas are telling them and everyone else—i.e. that their previous life’s work was for most part an unfortunate waste of time. And so they are rarely welcomed and typically resisted, pushed back into the darkness via aggression and ridicule. Although powerful, simple ideas aren’t eternal; they only possess elongated lifespans that often exceed their creator’s, their generation’s, or the millennia. Ultimately, they also suffer of death by exaggerated extension, and are replaced in bulk by other, simpler, siblings. As mortal men, however, we must admit we don’t have much better than radically abstract, powerful mental models lasting more than families, nations, and cultural traditions.
Each of us has one, or maybe a few, simple ideas that once interiorised have progressively influenced our perception of reality like infinitely potent acid. We might know their names, or we might not. In my arsenal of brutally simple yet outrageously powerful ideas stands a logical technique called reductio ad absurdum, or proof by contradiction. The reductio is a logical form of argument that resolves an open question by demonstrating that its validity would necessarily lead to absurd results. You would be surprised by how many claims, especially in complex environments like social sciences, this technique quite simply obliterates—an old analysis of Olympus’s bonding mechanisms here on DR is a good example.
In a bit more detail, a proof by contradiction works as follows:
Identify which logical proposition P is to be proven
Assume P to be false, i.e. its opposite ¬P to be true
Derive from the assumed truth of ¬P two opposite assertion, Q and ¬Q
Conclude the validity of its alternative, the original proposition P
One of the most famous examples of proof by contradiction is Euclide’s theorem about the infinity of prime numbers. The theorem goes like this:
P → "primes are more than any assigned multitude of primes"
¬P → "primes aren’t more than any assigned multitude of primes"—i.e. for a specific natural number n, there are no primes larger than n
Is ¬P true? Given that this would mean primes are finite let’s assume we can make a list of all primes {p(1), p(2), …, p(k)}; let P = p(1)*p(2)*…*p(k)be the product of all those primes, and Q = P + 1. Given that Q is by definition larger than P, it cannot be a prime, and hence it must be divisible by one of them, let’s say p(i). This means that both Q and P are divisible by p(i) and so should be their difference Q - P = 1, but this cannot be possible because 1 cannot be divisible by any prime. ¬P cannot be true
Given that ¬P is false, P must be true → The point concludes our proof; while it would have been extremely difficult to demonstrate the infinity of prime numbers, the impossibility of this infinity is way easier to demonstrate, yet exactly as powerful
The history of mathematics is full of those types of arguments, with Turing’s proof of the Entscheidungsproblem, and Gödel’s proofs of his incompleteness theorems maybe among the most prestigious examples. Today’s mathematicians and logicians believe we have plenty of those easy to check / difficult to solve (P ≠ NP) problems, although so far nobody has proven that such inequality holds—if you believe in your arguments you can apply to receive the 1 million USD grant here. We often engage in heated discussions of principle to demonstrate the wrongness of an argument, while most of the times it would be enough to show the absurdities implied by the argument itself.